Twin Paradox with Sinusoidal Speed Variation
Introduction
It has always seemed to me that the hardest part to swallow about the twin paradox is the “instantaneous” turnaround of the spaceship twin at his farthest distance from earth. The following treatment will analyze the total time observed by the spaceship twin when his speed profile is sinusoidal so that his turnaround(s) are smooth.
Equations
We will express the speed of light, c, in light years per year and designate a round trip that takes a total Tt=10 years (subscript t stands for trip). Of course, the distance traveled by the spaceship will be less than 10 light years because the ship’s speed varies sinusoidally as:
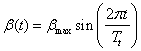
where, of course, the velocity, v, is:
![]()
We can determine the x coordinate at any time by integrating v(t):
![]()
where, for convenience, I have designated:
![]()
Obviously
![]()
so the total distance traveled is 6.36 light years.
The time observed by the space twin will be measured as the accumulated phase of a Doppler-shifted light wave she receives from earth. On earth the radian frequency, wc, (where subscript c stands for clock) of this signal is perfectly constant and the signal is expressed as
![]()
where E0 is the peak amplitude of the signal and, for better time resolution, we will assume that wc>>wt.
The space twin will see the frequency of the Earth signals
Doppler shifted by the factor:

Here the b(t) in the numerator represents the effect of the space twin’s present speed with respect to the wave-fronts (signals) already present at his position x(t). The factor
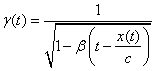
is the time-lagged Lorentz factor which represents the rate of the Earth clock as seen by the space twin at his present position. To understand how we got this time lag consider an event that happened on Earth at time tEvent. The information about this event is sent toward the space ship starting at time tEvent and its x position is:
![]()
From this equation we can solve for the time that this event is received by the space ship :
![]()
The event that we are considering here is that the space
ship has changed speed due to the sinusoidal velocity Vs.
time profile.
The total phase that the space twin observes up to time t is
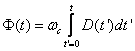
The following is a plot of both F(t) and wct Vs x(t):
![]()
![]()
![]()
![]()
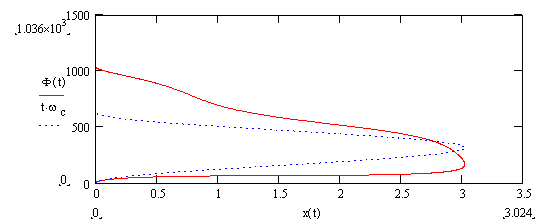
Figure 1:Phase accumulation
of a local clock(blue trace) and perceived phase accumulation of Earth clock as viewed from a space ship in
sinusoidal motion with a maximum speed, bc, of 0.95 c.
where wc was chosen so that earth emitted 10 cycles per year
and bmax was chosen as 0.95. The starting condition for both the earth
phase and the space phase is, of course, zero.
Note that that blue trace is symmetric with respect to outgoing and
incoming phase accumulation. However,
the red trace shows a dramatic increase in phase accumulation about one half of
the way through the trip and ¾ of the way through the trip. These locations are where the denominator of D(t) is quite small.
The
spaceship twin also has a clock identical to that of the earth twin and its
phase accumulation is thus necessarily
given by the blue trace in Figure 1. So the spaceship twin’s perception of the
earth twin’s phase (or time) accumulation ends up larger than his own local time
phase (or time) accumulation.
In order to
compare this time history to a profile more akin to the usual instantaneous
turnaround, I have also computed the time history for what I call a trapezoidal
b profile. That profile and result is
discussed and shown in the Appendix.
Conclusions
Although somewhat more mathematical than the usual instant-turnaround space trip analyses, I think that this treatment is much more convincing than the other-because sudden coordinate frame jumps are just really hard to grasp-to me they’re similar to sleight of hand.
Some may argue that I haven’t included the general relativistic effects of acceleration. With this speed profile, I can at least state that the peak acceleration can be made as small as desired simply by making the trip time longer.
Appendix: Trapezoidal b Profile
This b profile is piecewise continuous and each half forms a part of the perimeter of a trapezoid as shown in Figure A1:
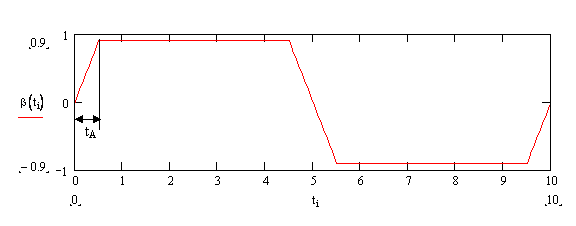
Figure A1: Trapezoidal b profile with
peak b value of 0.9 and acceleration time, tA, of
0.5 light years.
Using exactly the same equations as for the sinusoidal profile discussed above, we get the following plot of accumulated phases Vs position, x.
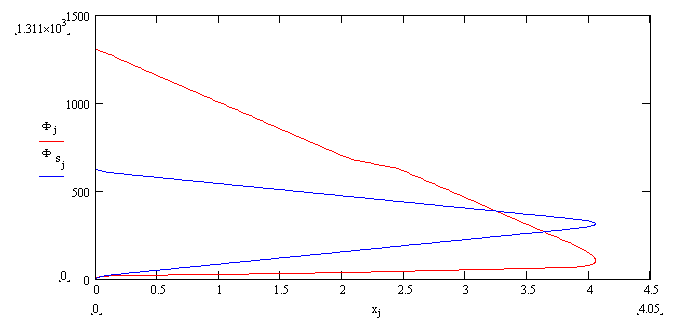

Figure A2: Phase accumulations (PA) Vs x for the
parameters shown in Figure A1. The red
trace is the PA that the space twin observes of an emitter based on Earth. The blue trace is the PA that the space twin
observes from his own identical local emitter.
![]()
![]()

Figure A3: Same PA as in Figure A2 but with more
aggressive b profile parameters. This time the peak b value is 0.99 and the tA
is only 0.2 light years. The first half
of the red trace is not visible because the observed Earth PA rate is so small
there.
Note that the ratio of Earth PA to local PA here is
considerably larger than that for the sinusoidal b profile. This is partly because a lower fraction of
the trip is spent at lower speeds while accelerating.